Pendule d'Atwood

Le pendule d'Atwood est un mécanisme qui ressemble un peu à une simple machine d'Atwood, si ce n'est que l'une des masses peut osciller dans un plan.

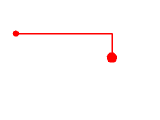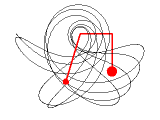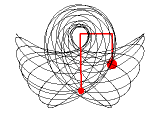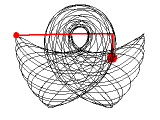
Le pendule d'Atwood possède deux degrés de liberté, la longueur du pendule r et l'angle θ. Son mouvement peut être décrit dans un espace des phases à quatre dimensions r, θ et leurs dérivées premières. La conservation de l'énergie limite le mouvement à un sous-espace à trois dimensions et il est possible d'imposer des restrictions supplémentaires au système.

Le hamiltonien de ce système s'écrit

${\displaystyle H(r,\theta )=T+V={\frac {1}{2}}M{\dot {r}}^{2}+{\frac {1}{2}}m({\dot {r}}^{2}+r^{2}{\dot {\theta }}^{2})+gr(M-m\cos(\theta )),}$

où g est l'accélération de la pesanteur, T et V étant respectivement l'énergie cinétique et l'énergie potentielle.

Les systèmes hamiltoniens peuvent être classés en systèmes intégrables et non-intégrables. Le pendule d'Atwood est intégrable dans le cas où le rapport de masse, M/m vaut 3. Pour de nombreuses autres valeurs de ce rapport de masse, le pendule d'Atwood adopte un mouvement chaotique.

Cet article est issu de wikipedia. Text licence: CC BY-SA 4.0, Des conditions supplémentaires peuvent s’appliquer aux fichiers multimédias.